Financial Literacy / Money from Credit Cards

Stoozing or How to Make Money from Credit Cards

by cash4nothing · October 28, 2021

This is it. This is a Big Man’s Game now. I mean this is the moment where my Free Money making methods are notching up a step and reaching a refinement level I am most comfortable with. Let me point out that this is an advanced way of making Free Money and neither a straight forward nor an intuitive one. It also demands for the money to be invested in the medium to long term to generate relevant profits. This is a technique that I have used systematically in the past reaping substantial rewards in the process (see I managed to accrue £22,750 from Free Money Sources Post). The absolute beauty of it is that I will not be using my own money to make free money but someone else’s.

How does it (Stoozing) work?

In a nutshell: I borrow money at no interest (or very low interest) and use it to invest it in a ‘safe’ proposition. I will be paying back the very minimum amounts as monthly instalments for the duration of the loan as well as the outstanding figure at the end of the loan lifespan. The idea is that all this time, the borrowed money has been producing profits from the investment portfolio. The Free Money comes from pocketing the difference in between these profits and the loan repayment amounts.

This technique is termed as Stoozing or the Fine Art of making free money by taking advantage of credit card offers. There are many variations of it, but the principle remains the same: use cheap or free money to collect free cash as a result of investing it in a no risk or low risk proposition.

Example: Borrow a £1,000 for a year and invest it earning an 8% interest

Iteration 1

Minimum monthly repayment: 0%

Cost of borrowing: £0

Let’s start with a simplified example: let’s say that I have managed to borrow £1,000 today and I will have to pay it back in a years’ time. Let’s also say that I am in a position to invest that money in a proposition returning 8% per year. One year down the line, I will be ending up with £1,080. At that moment, I pay the £1,000 loan back and I will be keeping the £80 investment profit for myself. I did not have to use any of my own money to earn the £80 so, theoretically, the Return on Investment (ROI) would be infinitum £80/£0. That sounds really good.

Iteration 1 Profit: £80 / Return On Investment (ROI): infinitum

Iteration 2

Minimum monthly repayment: 1% of outstanding amount

Cost of borrowing: £0

Let’s have another iteration and incorporate the fact that I will have to pay some of the borrowed money back over the course of the year. Let’s say 1% of the outstanding amount per month. So, in month 1, I am paying £10 back, and as a result, the outstanding amount will be £990; in month 2, I will be paying £9.90 back, and the outstanding amount will come to be £980.10. And so on over the 12 month period (see table below).

After 12 months, I would have repaid £105 and the outstanding debt would be £895. In the meantime, my £1,000 investment would have generated £80 in profit.

In summary:

Month	Outstanding	Payment	Accumulative
1	£1,000
2	£990	£10	£10
3	£980	£10	£20
4	£970	£10	£30
5	£961	£10	£39
6	£951	£10	£49
7	£941	£10	£59
8	£932	£9	£68
9	£923	£9	£77
10	£914	£9	£86
11	£904	£9	£96
12	£895	£9	£105

Once the debt is settled, I get to keep £185. I pay myself back £105 and keep £80 as Free Money Earnings.

I used £105 of my own money to generate £80.

Return on Investment: £80/£105 = 76%.

Iteration 2 Profit: £80 / ROI: 76%

Take your time to understand and digest these figures. A 76% return on investment. In other words, for every £1 of your own money, you produced 76 pence in profit. I do not know many investment propositions offering that level of return out there. I will welcome any suggestions as I am always eager to try new things.

Iteration 3

Minimum monthly repayment: 1% of outstanding amount

Cost of borrowing: £30 (3% of the Loan amount)

As a third iteration, and in real terms, there will be a cost involved in obtaining the loan, and that is the transaction fee. That is the cost that the lender will pass on to you for awarding the loan. Let’s say is 3% of the loan amount, or £30 based on our £1,000 example. The revised figures are as follows:

Month	Outstanding	Payment	Accumulative
1	£1,030
2	£1,020	£10	£10
3	£1,010	£10	£20
4	£999	£10	£31
5	£989	£10	£41
6	£980	£10	£50
7	£970	£10	£60
8	£960	£10	£70
9	£950	£10	£80
10	£941	£10	£89
11	£932	£9	£98
12	£922	£9	£108

In summary:

I have paid £108 but the outstanding debt is £922. My £1,000 investment generated £80 in profit.

Once the debt is settled, I get to keep £158. I pay myself back £108 and keep £50 as Free Money Earnings.

I used £108 of my own money to generate £50.

Return on Investment: £50/£108 = 46%.

Iteration 3 Profit: £50 / ROI: 46%

The fee obviously eats up into the profit and reduces the ROI by almost half, but nevertheless, it is still a phenomenal 46% Return on Investment. An opportunity too good to be missed.

Iteration 4

Minimum monthly repayment: 1% of outstanding amount

Cost of borrowing: £30 (3% of the Loan amount)

Loan Term: 24 months

As a fourth iteration, let’s assume that the duration of the loan is 24 months but the fee is only paid at the start.

Month	Outstanding	Payment	Accumulative
1	£1,030
2	£1,020	£10	£10
3	£1,010	£10	£20
4	£999	£10	£31
5	£989	£10	£41
6	£980	£10	£50
7	£970	£10	£60
8	£960	£10	£70
9	£950	£10	£80
10	£941	£10	£89
11	£932	£9	£98
12	£922	£9	£108
13	£913	£9	£117
14	£904	£9	£126
15	£895	£9	£135
16	£886	£9	£144
17	£877	£9	£153
18	£868	£9	£162
19	£860	£9	£170
20	£851	£9	£179
21	£842	£9	£188
22	£834	£8	£196
23	£826	£8	£204
24	£817	£8	£213

Revised summary:

I have paid £213 but the outstanding debt is £817. My £1,000 investment generated £166 in interests over two years.

Once the debt is settled, I get to keep £349. I pay myself back £213 and keep £136 as Free Money Earnings.

I used £213 of my own money to generate £136.

Return on Investment: £136/£213 = 64%.

Iteration 4 Profit: £136 / ROI: 64%

Why not use your own money to save £1,000 and invest it over the next 24 months?

Let’s use some numbers to answer that question. If I want to save and invest £1,000 over a 24 month period, I need to put £41.67 aside every month into my 8% investment pot. 8% per year translates into 0.64% return per month. The table below summarises the projected returns.

Month	Saving+Return
24	£41.67
23	£41.94
22	£42.21
21	£42.48
20	£42.75
19	£43.02
18	£43.30
17	£43.57
16	£43.85
15	£44.13
14	£44.42
13	£44.70
12	£44.99
11	£45.27
10	£45.56
9	£45.85
8	£46.15
7	£46.44
6	£46.74
5	£47.04
4	£47.34
3	£47.64
2	£47.95
1	£48.26
Total	£1,077.26

I will use £1,000 of my own money to generate £77.26 profit.

Return on Investment: £77/£1,000 = 7.73%.

£1,000 of my own money at 8% – Profit: £77 / ROI: 7.7%

The disadvantage is obvious: in comparison to using other people’s money, I will be getting half the profit and 1/8 of the ROI. Not only that, I will need to put down £1,000 of my own money instead of £213. The opportunity cost is huge. By using someone else’s money, I am making use of leverage to multiply the returns of my own limited funds.

Allow me now to present this idea to you: what if I used £1,000 of my own money to service the minimum payments of a £5,000 borrowing over 24 months (£213 x 5 = £1,065)?

£1,000 of my own money to pay a £5,000 loan invested at 8% over 24 months – Profit: £680 / ROI: 64%

I hope that this sufficiently exemplifies the power of leverage. I do not intend to go for it just yet. As with all things, I start small and then progress to scale up subject to a well defined criteria (see ‘How much to borrow?’ box below).

Three obvious conclusions from my Investment Indicators

The lowest the cost of borrowing, the highest the Profit and the highest the ROI.
The longest the time span, the highest the Profit and the highest the ROI.
The lowest the monthly payment, the highest the ROI.

My job hence, is to find the right money lending offer with the lowest setup fee, the lowest monthly payment and the longest time span.

How much to borrow?

The next obvious question is: assuming I have credit at my disposal, how much should I borrow? The following red lines summarize my personal criteria.

I never borrow more than my actual savings. All my liabilities need to be covered by an equal amount in savings.
The projected pay back instalments need to be covered by cash reserves for the duration of the loan.

To finish my example off. If I was to borrow a £1,000 for 24 months paying back a 1% of the outstanding loan per month, I would need to have £1,000 in savings and £213 in cash reserves.

The right time for me to start Stoozing back again

Why haven’t I used Stoozing from the outset? Well. In my book, there are two basic requirements to be met:

I needed a certain amount of money in terms of cash reserves sufficient to cover monthly payments.
The right opportunity/promotion needs to came by to make a business case for me as regards to Return on Investment as well as meeting the outlined self-imposed criteria.

Barclaycard 24 Month Balance Transfer Offer

The stars aligned for me in early September 2021: I had £1,000 in savings, I also happened to have a Pot of £240 Free Money as Cash Reserves, and I received a very appealing offer from Barclaycard in my inbox.

What are the conditions of the Barclaycard offer?

0% Balance Transfer until 1^st September 2023: 24 month Loan duration

Balance Transfer fee: 3.4%

Minimum repayments: 2.75% of the outstanding balance

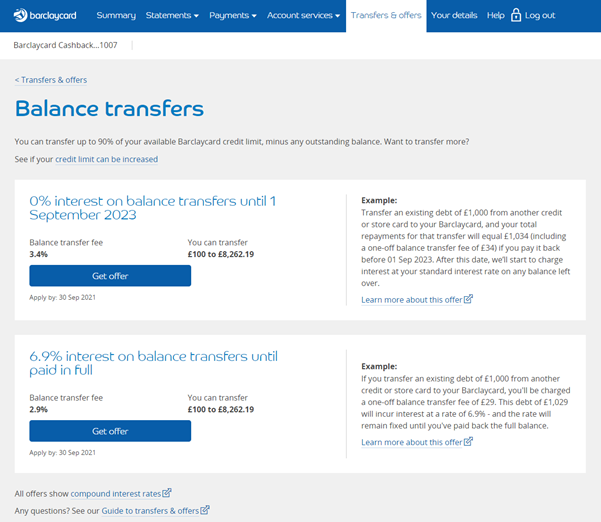
What do the projection numbers throw if I was to invest a £1,000 at an expected 8% return over 24 months?

Month	Outstanding	Payment	Accumulative
1	£1,034
2	£1,006	£28	£28
3	£978	£28	£56
4	£951	£27	£83
5	£925	£26	£109
6	£899	£25	£135
7	£875	£25	£159
8	£851	£24	£183
9	£827	£23	£207
10	£805	£23	£229
11	£782	£22	£252
12	£761	£22	£273
13	£740	£21	£294
14	£720	£20	£314
15	£700	£20	£334
16	£681	£19	£353
17	£662	£19	£372
18	£644	£18	£390
19	£626	£18	£408
20	£609	£17	£425
21	£592	£17	£442
22	£576	£16	£458
23	£560	£16	£474
24	£544	£15	£490

I will pay £490 and the outstanding debt will be £544. My investment will be generating £166 in interest payments.

Once the debt is settled, I get to keep £622 (£456 + £166). I will pay myself back £490. Free Money Profits: £132

I will use £490 of my own money to generate £132 profit.

Return on Investment: £132/£490 = 27%.

Barclaycard Stoozing Projection at 8% Interest over 24 months

Profit: £132 / ROI 27%

Frequently Asked Questions (FAQs)

Do I have sufficient money in cash reserves to cover the £490 cash flows requirements?

As it stands, £240 will be sufficient for the first 10 months of outgoings. However, I will need to come up with an additional £250 for the next 24 months. Knowing that I have a rule in place by which I allocate 10% of my Free Money takings towards the Cash Reserves Pot, I am going to have to deliver a minimum of £2,500 in Free Money Earnings over the next 24 months. Wow. The task ahead is set for me. I just need to figure out how to come up with the goods.

What happens at the end of the promotional offer?

I have two basic options:

Repay the outstanding amount of £544.
Transfer the Balance of £544 to another credit card and initiate a new cycle.

My definite pick by default is always number 2.

Can I get a better offer?

Currently, there are credit card balance transfer offers with a 0% fee and for up 30 months. My constraint is the amount of credit I have available to my name and my Credit Score. After running a soft credit check on my record prior to applying, I am finding that I do not qualify for most of them at this moment in time. So rather than taking my chances and applying for fresh credit at a risk of eroding my Credit Score, I will settle for a competitive enough offer from any of the credit cards I hold providing the ROI is appealing enough and within my parameters. The offer from Barclaycard is a 3.5 out of 5. Good enough for me.

Why use cash reserves and not my own money?

I also do use my own money to make free money from credit cards, but that is a separate chapter of my personal finances away from my Free Money Earning activities. This Blog is about how I gather Free Money and how I invest it into a Savings Fund. On this basis, I am only allowing myself to use the Free Money from the Cash Reserves Pot to support the Credit Card cash flows liabilities. My view is that I am transforming Cash Reserves (not generating any returns at the moment) into solid investments and using them as a leveraging tool in the process to pay back the borrowed capital.

How do I move the money into my Bank Account from a Credit Card Balance Transfer offer?

For that I will use a small trick of the trade. I will charge a £1,000 into my spending credit card from my Revolut Account and from then, I will send the money to my current bank account. After that, I will balance transfer the £1,000 from my spending credit card to the donor credit card. Job done.

How will the £1,000 borrowing be deployed?

The £1,000 has been transferred into my bank account. Where is the money going to be invested? No suprises really. I will remain coherent and in alignment with my 60/30 Investment Strategy: £665 IFISA and £335 S&S ISA. My expected tax free returns will fluctuate in between 7% and 8% per year.

How will the Free Cash Fund Value be affected?

I will need to account for liabilities when calculating the Free Cash Fund Value from now on. I will also have to keep a record of Cash Flows as regards to the Cash Reserves element:

FCF Value on September 2021 £3,596-£1,034 (Credit Card Borrowings) = £2,562

Cash Reserves adjustment: £240-£28 (first Credit Card Payment) = £212

I will be making a mental note: if no further contributions into the Fund were being made and assuming an average growth of 8% per year, I will be expecting £2,988 by September 2023. I will set a gateway in my calendar to check on my projection.